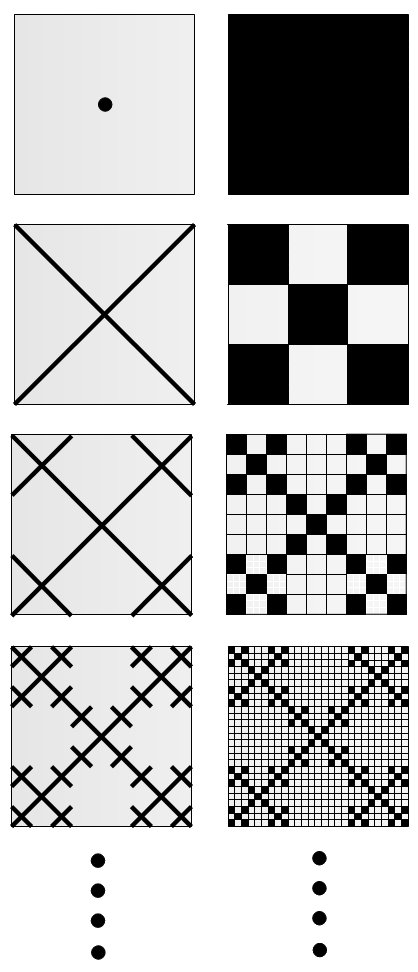
 Fractal objects appear similar at different scales. There are many properties of an object that are candidates for similar appearance and in the case of two and three-d objects, these are scaled versions of the same two and three-d geometries. But changes in scale also occur in time: a time series over say minutes often has the same geometric shape and statistical properties as that over days or over seconds. The key signature of scaling is an algebraic form that appears the same if we literally scale its metric. The only function that satisfies this criterion is a power law. If, say, the size of an object called P is a function of the metric length L at which it is observed and the relation is given as P=k(L^a), then if we scale the metric length L by a factor q, we get P’=k([qL]^a) which simplifies to P’=k(q^a)(L^a)=(q^a)P.
Fractal objects appear similar at different scales. There are many properties of an object that are candidates for similar appearance and in the case of two and three-d objects, these are scaled versions of the same two and three-d geometries. But changes in scale also occur in time: a time series over say minutes often has the same geometric shape and statistical properties as that over days or over seconds. The key signature of scaling is an algebraic form that appears the same if we literally scale its metric. The only function that satisfies this criterion is a power law. If, say, the size of an object called P is a function of the metric length L at which it is observed and the relation is given as P=k(L^a), then if we scale the metric length L by a factor q, we get P’=k([qL]^a) which simplifies to P’=k(q^a)(L^a)=(q^a)P.
In short, P’ is a simple linear function of P and in this sense, we can say it has the same appearance or form. The scaling factor q simply scales the object up or down in a linear, hence similar way
In what follows, we will explore how scaling pertains to the geometry and morphology of cities and buildings.
UNDER DEVELOPMENT …..
